Unistellar eVscope: Field of View Comparison with the Atik Infinity Camera

Introduction

Since I bought an Atik Infinity camera in "anticipation" of or in preparation for the eVscope, which was promised to be delivered starting in November 2018, I was, of course, curious finding out, whether both solutions provide a similar image quality and field of view (or how I can achieve this).

Note: A first hint at the differences in image quality can be found on page Atik Infinity Colour Camera versus eVscope - Photo Comparison.

First of all, the sensors differ in that the IMX224 is a CMOS sensor, and the ICX825 used in the Atik Infinity is a traditional CCD sensor. Secondly, they differ in their size: The diagonal of the ICX825 is almost twice as large (11 mm) as that of the IMX224 in the eVscope (5.5 or 6.1 mm, depending on the type of application). Both sensors have similar pixel counts (1392 x 1040 versus 1305 x 977/1280 x 960*). Accordingly, the (square) pixels of the ICX825 are about three times as large as those of the IMX224 (6.45 μm vs. 3.75 μm edge length). What all this means in practice, I will probably find out only in the course of time ...

*) The eVscope uses the resolution of 1280 x 960 pixels.

Field of View ...

In its FAQ, Unistellar states a field of view of ~30' (about 0.5°, about the size of the diameters of sun and moon) for the eVscope, moreover they state that a pixel corresponds to a viewing angle of about 1.7". Using the field of view calculator on Astronomy.tools, I got a resolution of 1.72" and will use this in my calculations where appropriate.

When I multiply 1.72" by 1 1280 pixels, I get 36.7' (or 0.61°), which is considerably more than the stated 30'.

Below, I use three more approaches to calculating the (true) field of view:

from the focal length of the telescope and the sensor size (the longer edge)
using the field of view calculator on Astronomy.tools
using the diameter of the moon on an eVscope photo
using Stellarium from the focal length of the telescope and the sensor data

Field of View from the Focal Length of the Telescope and the Sensor Size

For the field of view of a camera attached to a telescope, Oden lists the following (approximative) formula:

Arc (°) = edge length of the sensor (mm) / focal length of the telescope (mm) * (180° / pi) (180/pi = 57.3...)

When I use 1280 pixels, I arrive at an edge length of 4.8 mm given a pixel size of 3,75 µm; the eVscope has a focal length of 450 mm. If I insert both values into the formula, I arrive at:

Arc (°) = 4.8 (mm) / 450 (mm) * (180° / pi) = 0.61° = 36.7'

This value corresponds to what I got above for 1280 x 960 pixels.

Field of View Calculator on Astronomy.tools

I entered the following values into the field of view calculator on Astronomy.tools:

Focal length : 450 mm, aperture: 114 mm
Pixels: 1280 x 960, pixel size: 3.75 µm x 3.75 µm

Results:

Field of view : 0.61° x 0.46° (36.7' x 27.6')
Resolution: 1.72" x 1.72"

These are more or less the results, that I got using the formula given by Oden and the values given by Unistellar. In addition, I prefer the resolution given by this calculator to the value given by Unistellar, because it is one decimal more precise.

Estimation of the Field of View Based on the Diameter of the Moon

In May 2020, I photographed the almost full moon with the eVscope. Based on the photo I estimated its diameter to be 1180 pixels, which corresponds to a viewing angle of about 30' (according to Wikipedia 29.4' to 33.5'). Since the moon was quite big at that date and I was not ablt to find its angle of view, I assumed a value of 33' for it.

Estimation of the eVscope field of view: Moon approx. 1180 pixel diameter = 33'
33': 1280 pixels = 35.8'; 960 = 26.8'

This is slightly less than calculated, but also involves certain inaccuracies. Considering this, the estimated value agrees quite well with the calculated values.

Field of View from the Focal Length of the Telescope and the Sensor Data in Stellarium

In summer 2020, I entered the data of the eVscope and its sensor into Stellarium:

Focal length : 450 mm, aperture: 112 mm (same FOV as for 114 mm!)
Pixels: 1280 x 960, pixel size: 3.75 µm x 3.75 µm

Results:

Field of view : 36.67' x 27.5'
Resolution: 1.72" x 1.72"

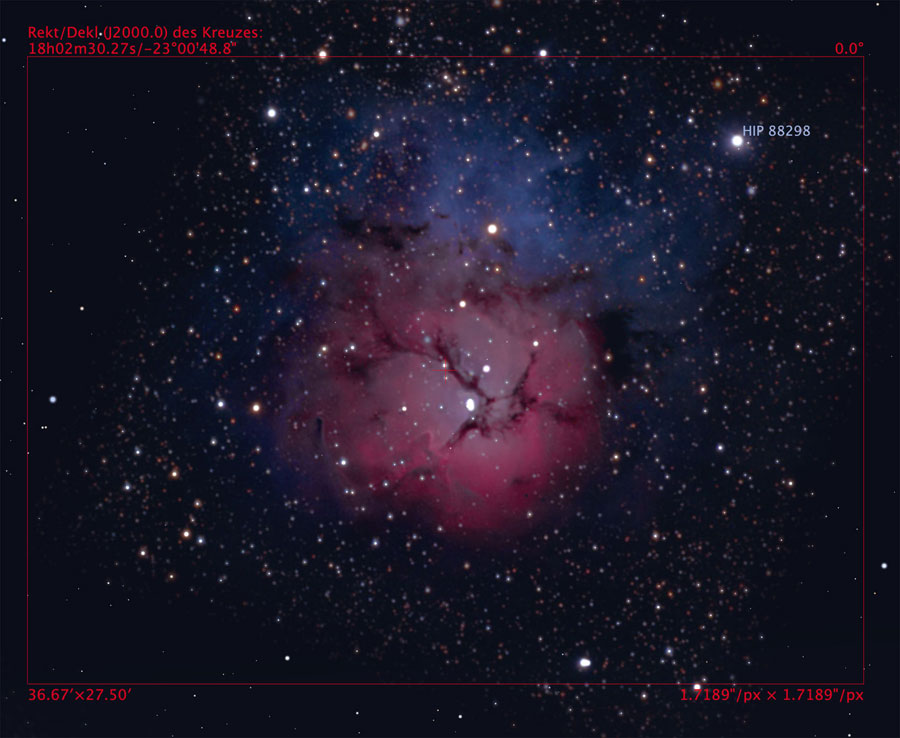
For illustration, I made a comparison between an eVscope photo and the display in Stellarium using the Trifid Nebula M 20:


Bildfeldanzeige für das eVscope in Stellarium mit M 20 (Trifidnebel)		eVscope-Foto von M 20 (Trifidnebel)

Please note that I show a larger section on the left because of the frame labelling in Stellarium. All in all, the correspondence seems to be quite good. It should be noted that many DSO are shown in Stellarium as photos with a rather small section. At least, you can see that these objects fit into the eVscope's field of view...

Atik Infinity

Atik provides the following formula (it is an adapted version of the formula provided by Oden and used above) for calculating the arc (in seconds) per pixel of the Atik Infinity camera:

pixel size (µm) / focal length (mm) * 206,3 = 6,45 / focal length * 206,3 = 1330,6 / focal length

For arriving at the edge length of the sensor, the pixel size has to be multiplied by the respective number of pixels for that edge (I arrive at 9 mm). When I insert into this formula the data for a telescope with a focal length of 450 mm (e.g. a f/4 Newtonian tube with an aperture of 114 mm), as it is used for the eVscope, I arrive at a field of view of 1.14° (68.6'), which is fairly similar to what I get for a 9 mm Plössl eyepiece, which achieves a magnification of 50 x. A telescope with a focal length of 500 mm (e.g. a f/4.38 Newtonian tube with an aperture of 114 mm) arrives at more or less identical values as a 9 mm Plössl eyepiece, that is, at a field of view of about one degree.

Of course, other focal lengths of the telescope would lead to different values for the field of view. Identical fields of view for both sensors would be obtained if the focal lengths of the telescopes used have the same ratio as the horizontal edge lengths of the sensors:

eVscope: 4.8/450 = 0.0167
Atik Infinity: 9/x * 450/4.8 => x = 9/4.8*450 = 843.75, that is, about 750...900 mm

A telescope with an actual or shortened / extended focal length of about 750 to 900 mm would therefore have a similar field of view as the eVscope. Differences arise from the different aperture ratios and light gathering powers of the telescopes used, as well as the differences in the photosensitivity and the noise behavior of the two sensors. The sensor in the eVscope has only one third of the area of the Atik Infinity sensor. At similar pixel counts, the pixels are just about one-third the size, which means that they only receive 1/3 of the light, making them about 1.5 f-stops less sensitive than in the Atik Infinity sensor. On the other hand, the eVscope has an aperture ratio of f/4, and that is crucial for the exposure time. It still remains to be clarified, what the consequences of all these differences are...

Comparison eVscope, Atik Infinity, Stellina, and Hiuni

Finally, a comparison table (borrowed from a different page on this site), which includes the Stellina telescope from Vaonis and the Hiuni telescope, and shows how the different sensors, and for the Atik Infinity camera, the different telescopes, affect the field of view and resolution.

Telescope/Camera >	Hiuni	Stellina	eVscope	Infinity
Manufacturer	Bosma	Vaonis	Unistellar	Atik
Telescope (AI)	Custom, Cassegrain	Custom, Refractor	Custom, Newton	6" Newton Explorer 150PDS, StarBlast 6, ...	5" Newton Heritage P130, Sky Prodigy 130, ...	4.5" Newton Heritage 114N, StarBlast 114, ...	4.5" Newton StarBlast 4.5, ...	8" Schmidt-Cassegrain C8 with f/6.3 reducer
Manufacturer (AI)				SkyWatcher, Orion	Sky-Watcher, Celestron	Sky-Watcher, Orion	Orion	Celestron
Focal Length	1524 mm	400 mm	450 mm	750 mm	650 mm	500 mm	450 mm	1280 mm
Aperture	152.4 mm	80 mm	112 mm	150 mm	130 mm	114 mm	114 mm	203 mm
Aperture Ratio	f/10	f/5	f/4	f/5	f/5	f/4.5	f/4	f/6.3
Resolving Power (Dawes)+	0.79"§/0.75"*	1.45"*	1.02"*	0.77"*	0.89"*	1.02"*	1.02"*	0.57"*
Resolving Power (Rayleigh)++	0.92"§	1.73"	1.12"	0.92"	1.06"	1.12"	1.12"	0.68"
Sensor	Aptina MT9M001-C/M	Sony IMX178	Sony IMX224	Sony ICX825
Sensor Type	CMOS	CMOS	CMOS	CCD
Pixel Resolution	1280 x 1024	3096 x 2080	1280 x 960	1392 x 1040
Pixel Size	5.2 µm x 5.2 µm	2.4 µm x 2.4 µm	3.75 µm x 3.75 µm	6.45 µm x 6.45 µm
Resolution H	0.27°/16.2' 10.3° (finder)	1° (1.06°/63.6'*)	~30' (0.5°) (0.61°/36.7'*)	0.69°/41.16'**	0.79°/47.49'**	1.03°/61.74'**	1.14°/68.60'**	0.40°/24.12'**
Resolution V	0.2°*	0.7° (0.71°*)	0.46°/27.6'*	0.51°*	0.59°*	0.77°*	0.85°*	0.30°*
Resolution per Pixel	0.7"*	1.24"*	1.72"*	1.77"**	2.05"**	2.66"**	2.96"**	1.04"**

*) Calculated with Astronomy.tools; **) my own calculations, verified with Astronomy.tools
+) also calculated as 114/aperture; ++) calculated as 138/aperture; § given by manufacturer

Conclusions

The eVscope uses the sensor with 1280 x 960 pixels (a certain sensor mode) and offers a calculatecd resolution of 36.7' or 0.61° (pixel resolution is 1.72"). The Atik Infinity uses 1392 x 1040 pixels, and its pixel resolution and thus, the field of view, depends on the focal length of the telescope used. A focal length of about 850 mm would come close to the eVscope's field of view.

The Vaonis Stellina telescope has a field of view that is almost twice as large as that of the eVscope, making it more suitable for larger DSOs. The 4 times higher sensor resolution, however, is less clearly reflected in the reproduction of details: a 15' large object (e.g. M 13 in Hercules) extends over 523 pixels with the eVscope and over 726 pixels with the Stellina; this is a factor of almost 1.4 and not earth-shattering... In addition to the number of pixels, the "quality" of the pixels (noise behavior etc.) is also important, which I cannot say anything about at the moment, except for that the pixels of the eVscope sensor are significantly larger than those of the Stellina sensor (3.75 µm compared to 2.4 µm).

The Hiuni telescope is better suited to observing planets and the moon than DSOs. Even the large aperture and the large pixels the maximum magnitude is only 12.8 mag. The field of view covers only half of the moon! But maybe the Hiuni can play to its strengths with some smaller DSOs (e.g. globular clusters, Ring Nebula M 57)... Note that an object of 15' like the Hercules Cluster M 13 nearly covers the complete width of the view of the Huini telescope (and more than its height). Thus, the Hiuni will offer about twice the number of pixels for this object than the eVscope.

There is one more thing that strikes me, but I do not have the knowledge to understand what this really means. For all the solutions shown for the Atik Infinity, the telescope resolves more than 2 times better (Dawes criterion) than the pixel resolution of the sensor. So you might say that these telescope tubes are "oversized" for the camera. The eVscope still has a factor of almost 1.7, but Stellina's and Hiuni's sensor resolve better than the telescopes. One might say that their sensors are "oversized" and that the telescopes cannot keep up with it. What that means in practice, I regrettably cannot tell you at the moment...